It is seen that these terms will need to produce another commutative geometry and algebra for this type of transmórfica physical and philosophical topology.
That is, both the graphs, groups and subgroups, paths, sets of vertices and edges, matrix and adjacency matrix,
The Graceli path determines that the return path is different from way back, even being the same.
The number of nodes in a rotation system and proportional to the number of rotation. Whenever there is a connection between two vertices and edges systems, which may be one of the two rotating.
A rotation system with all the colors have one color.
Theorem network.
Always when both a parallel and transversal connection between points of two or more types of interconnected nodes will be points where these forms are in rotation or in translation.
The edges tend to change shapes as distance and speed.
A graph edges passes to grade the slope edges as rotations and distances.
Up and then down a mountain is different from down and then up.
That is, a physical action logic composed.
Or of a different quality and time and space, giving a philosophical diverse logical and physical sense between b and b.
ie we have another area for a commutative algebra for addition and multiplication.
physical and philosophical geometry.
Imagine movements and variations and movements, interactions, types transformations, etc. and references forming part of a system of slopes and topological edges.
And with intensities, densities, colors, porosities, as topological categories that has action as aspects as well as edges.
Where the kit may be any of those elements and its variables.
In the case of the colors can make color combinations.
relativist commutative geometry.
A physical geometry or philosophy commutative a + b ≠ b + a, and b * ≠ b * a.
Imagine the color red on the green, it has a different training is over.
The same with the favorable or contrary wind, or even inertia. If one hits the front car are thrown forward, and if knocked back will be felt the jolt back.
Or even a donkey pulling a cart in a plane at the speed x is different if a slope, in this case the wagon that impure ass.
An observer inside the cart will have different references about who pulls or is impurado. In other words, it is relativistic.
There are many other examples that may be cited.
Imagine a system of lugs which are interconnected in a central point to various other peripheral and other peripheral such that only interconnects together.
And ranging interconnecting and disconnecting at all times in relation to a disconnection of interconnection and flows.
And it has other peripheral tracks are interconnected on and off, that is, these terms have isomorphic transmórfico system state variable conditions and back and forth [streams], that is, these terms have a variable set system. And that can be anything as the variables in which they are.
Imagine an octopus that moves with flows in its tentacles.
Or even a jellyfish with their movements flows inside out, according to the position where it forms have x, y, k, n ...
That is, while the isomorphic topology if you have an edge system, sheds and paths with directions, here comes other agents such as dynamics, interconnections, n-dimensions, and also observers positions where reality changes according to the position of each one of them.
And they become part of other categories of sets, numbers and also morphism.
Graceli topology edges and slopes. Transmorfismo.
Imagine strings being balanced in space, or even whips, where the edges are the strings become points [aspects], where each movement produces displacements and changes in tangents and sine and cosine between the edges and slopes.
The is, we have a transmorfismo regarding the position of the strings and their movements, making an n-dimensional transmorfismo.
That is, it can produce other edges in relation to points on the string, or between defined points, parallel, or transverse.
For, if numbered points can be close points, or Cross Crusaders.
And since the ropes can be also more than one, two, three and n-cords.
In this topology the edges [strings] turn into strands, and the edges are also links.
sexta-feira, 8 de abril de 2016
Vê-se que nestes termos se precisa produzir
outra geometria comutativa e álgebra para este tipo de topologia transmórfica
física e filosófica.
Ou seja, tanto os grafos, grupos e subgrupos,
caminhos, conjuntos, de vértices e arestas, de matriz e matriz de adjacência,
O caminho de Graceli determina que o caminho de
ida é diferente do caminho de volta, mesmo sendo o mesmo.
O número de nós num sistema de rotação e
proporcional ao número de rotação. Sempre quando houver uma interligação entre
dois sistemas de vértices e arestas, onde pode ser um só dos dois em rotação.
Num sistema de rotação com todas as cores se
terá uma só cor.
Teorema da rede.
Sempre quando estiver uma ligação tanto paralela
quanto transversal entre pontos de dois ou mais tipos de pontos interligados se
terá nós quando estas formas estiverem em rotação, ou mesmo em translação.
As arestas tendem a mudar de formas conforme
distância e rotação.
Um grafo de arestas passa a ter grau nas
arestas de inclinação conforme as rotações e distâncias.
Subir e
depois descer uma montanha é diferente de descer e depois subir.
Ou seja, é uma lógica de ação física composta.
Ou mesmo de uma qualidade e tempo e espaços
diferentes, dando um sentido físico filosófico e lógico diverso entre a b, e b a.
ou seja, temos outra vertente para uma álgebra comutativa para adição e multiplicação.
quinta-feira, 7 de abril de 2016
Geometria física e filosófica.
Imagine movimentos e variações e deslocamentos,
interações, tipos transformações, etc. e
referenciais fazendo parte de um sistema de vertentes e arestas topológicas.
E também com intensidades, densidades, cores,
porosidades, como categorias topológicas que tem ação como vertentes e também
como arestas.
Onde o conjunto pode ser qualquer destes
elementos e suas variáveis.
No caso das cores se pode fazer combinações de
cores.
Geometria comutativa relativista.
Numa geometria física ou filosofia comutativa a
+ b ≠ b+a, e a *b ≠ b*a.
Imagine a cor vermelha sobre a verde, se tem
uma formação diferente de for ao contrário.
O mesmo com o sentido favorável ou contrário do
vento, ou mesmo da inércia. Se um carro bate de frente somos jogados para
frente, e se for batido atrás será sentido o tranco para trás.
Ou mesmo um burro que puxa uma carroça num
plano na velocidade x, é diferente se for numa ladeira, neste caso a carroça
que impura o burro.
Um observador dentro da carroça terá
referencias diferentes em relação a quem puxa ou é impurado. Ou seja, é
relativista.
Existem vários outros exemplos que podem ser
citados.
Imagine um sistema de arestas que se interligam
de um ponto central para varias outras periféricas, e destes outra periferia
que apenas se interliga entre si.
E que variam se interligando e se desligando a
todo momento em relação a um fluxos de interligação e desligamento.
E que se tem outros faixas periféricas que se
interligam e desligam, ou seja, temos nestes termos um sistema isomórfico
transmórfico de estados de condições variadas e de idas e voltas [ fluxos], ou
seja, nestes termos temos um sistema de conjunto variável. E que pode ser
qualquer coisa conforme as variáveis em que se encontram.
Imagine um polvo que se movimenta com fluxos em
seus tentáculos.
Ou mesmo uma água-viva com seus movimentos de
fluxos que parte de dentro para fora, onde conforme a posição se tem formas x,
y, k, n...
Ou seja, enquanto na topologia isomorfa se tem
um sistema de arestas, vertentes e caminhos com direções, aqui se entra outros
agentes, como dinâmicas, interligações, n-dimensões, e também posições de
observadores, onde a realidade muda conforme a posição de cada um deles.
E que eles passam a fazer parte de outros tipos
de categorias de conjuntos, de números e também de morfismo.
Topologia Graceli de arestas e vertentes. Transmorfismo.
Imagine cordas sendo balançadas no espaço, ou mesmo chicotes, onde as arestas que são as cordas se transformam em pontos
[vertentes], onde cada movimento produz deslocamentos e variações nas tangentes
e seno e cosseno entre as arestas e vertentes.
O seja, temos um transmorfismo em relação a
posição das cordas e de seus movimentos, tornando um transmorfismo
n-dimensional.
Ou seja, possa se produzir outras arestas em
relação a pontos das cordas, ou seja, entre pontos delimitados, paralelos, ou
mesmo transversais.
Pois, se for numerados os pontos pode ser
pontos próximos, ou mesmo cruzados transversais.
E sendo que também as cordas podem ser mais de
uma, de duas, de três e n-cordas.
Nesta topologia as arestas [cordas] se
transformam em vertentes, e as arestas também são de ligações.
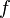 [ C m a [pa] [T]] =
[ C m a [pa] [T]] =
Cordas, movimentos, arestas, posições de
arestas.T = transmórfica = transcendente.
onde  é em qualquer posição função holomórfica não nula.
é em qualquer posição função holomórfica não nula.
 é em qualquer posição função holomórfica não nula.
é em qualquer posição função holomórfica não nula.
Assinar:
Comentários (Atom)